A discrete function $f$ on a discrete surface is just a map

$f:V\to \mathbb{R}$.

The derivative of $f$ is the function

$df:E\to \mathbb{R}$

$df(e)=f(\text{end}(e))-f(\text{start}(e))$.

$df$ has the property

$df\circ \rho =-df$

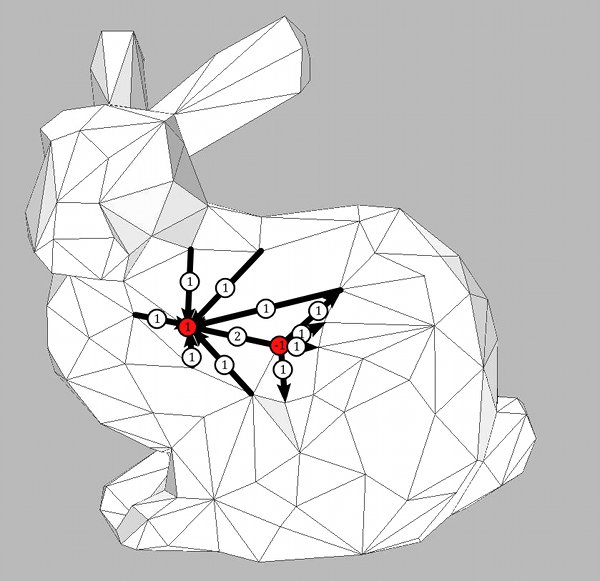
One way to visualize simple functions is to write $f(v)$ on every vertex $v$ where $f(v)\ne 0$. To visualize the derivative one can choose for each unoriented edge $ϵ$ on which $df$ does not vanish that oriented edge $e\in ϵ$ for which $df(e)>0$. Then one can draw $e$ as an arrow and indicate the “strength” of $df$ on $e$ by writing the number $df(e)$ on the arrow. Of course one could also choose for every $ϵ$ an arbitrary orientation $e\in ϵ$, draw the arrow and indicate $df(e)$ by writing the (now possibleý negative) number $df(e)$ on the arrow. The above convention has the advantage that the resulting picture almost looks like the gradient vector field of a smooth function on a smooth surface.